Median of Continuous Random Variable 1 2 Minimized
Show that if Y is a continuous random variable. with pdf f(y) then E|Y−c| is minimized at c=median(Y). Recall that a median mm satisfies ∫m−∞f(y)d(y)=∫∞mf(y)d(y)=1/2. Hints: a) Dropping the absolute value sign, rewrite E|Y−c| as a sum of two integrals. b) Integrate the sum w.r.t. mm and set the derivative equal to zero. c) Solve the resulting equation for mm. Don't forget to verify that the solution indeed gives the minimum the integral ranges from -oo to m and m to oo
Related Question
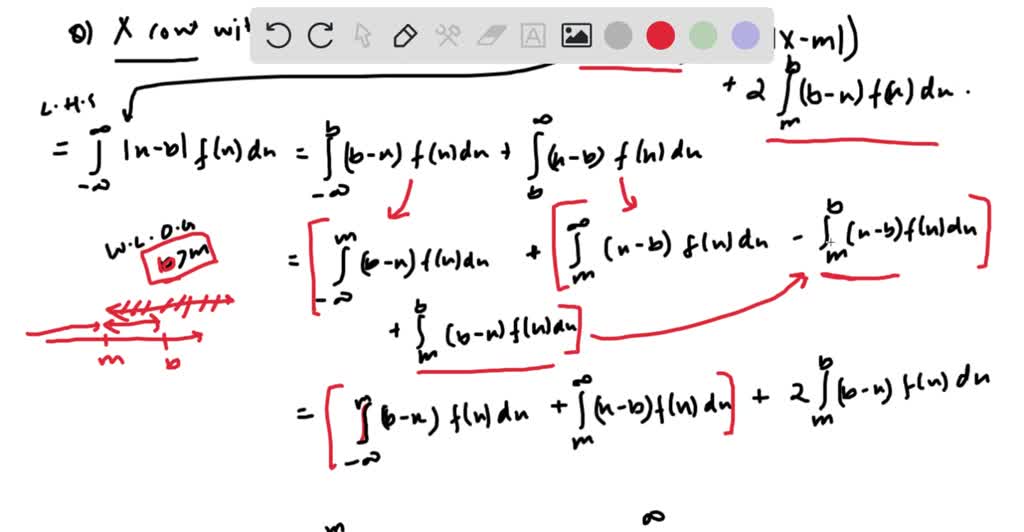
Let $X$ be a random variable of the continuous type that has pdf $f(x)$. If $m$ is the unique median of the distribution of $X$ and $b$ is a real constant, show that $$ E(|X-b|)=E(|X-m|)+2 \int_{m}^{b}(b-x) f(x) d x $$ provided that the expectations exist. For what value of $b$ is $E(|X-b|)$ a minimum?
Discussion
You must be signed in to discuss.
Video Transcript
So in this question we are given that X is a continuous random variable with unique comedian and and we need to show this equality of you and to see that expectation of X minus some constant be will be seen as expectation of X around him plus listen to them. So the way we'll do it is we will take this integral and using the definition of it, this will be integral minus infinity to infinity. Oh more expense P F of X dx And we'll break into two parts from manage invented from minus infinity will be and from beetle infinity. So next we'll assume that without loss of any generality B is greater than him. So this over here can again be broken down from minus infinity to em And then from empty in three D sorry from mt lb b minus six because so okay, in this part uh huh models X -B will be B -6. So and in this part more of expense. We will be expensive. The nexus between beetle and committee. So this part can be broken down as from M to infinity but we are taking a bigger interval. So we subtract from him to be the same individual. So the situation is like this we have the line you're saying I miss less than B. So for the first interval we're taking from minus infinity Tell em and then empty will be and for the second integral we are taking from empty infinity And from there we're subtracting this integral. So sorry from there. We're subtracting this part. So next uh so we can um so take this one and this one and take them to be together. We can yeah began absorb the negative sign by observe the negative side instead. And these two intervals will be same. So this will come out as two times empty B b minus X. F. Of X dx observing the negatives. And inside what will remain is these two parts minus infinity to them, B minus X for F of X. Dx. And I'm telling vanity explains B F of X. P X. Not that this quantity will have been equal to expectation of more expensive him if instead of BV had em. So then this country would have been same as this. So we will try to get him inside. So for the first part inside the bracket week add and subtract him. So we'll get a -6. And 2nd part of also will add and subtract them so that we get x minus M. Sylvia. So next we'll take this together. So first we want a minus X. This part will take together and in the second part we want m minus x minus M. So this part will take together. So these two will come out as expectation of more X men is um and ones which will remain is b minus M. Times mom integral minus ability to L. M F of X Dx. Yes your this part will be and minus B. Times into the empty infinity F of X dx. Since m is the unique median this country will be equal to have and this quantity will be equal to half, so feet get half times b minus um plus half times n minus B, Which is equal to zero. So Orel interval will be a little expression will be expectation of x minus B will be equal to this expectation of explains him. Plus this too two times until B b minus X for x dx. So this will be oral expression, which we wanted to show.
pattersonfisittests.blogspot.com
Source: https://www.numerade.com/ask/question/show-that-if-y-is-a-continuous-random-variable-with-pdf-fy-then-eyc-is-minimized-at-cmediany-recall-that-a-median-mm-satisfies-mfydymfydy12-hints-a-dropping-the-absolute-value-sign-rewrite-e-77433/

0 Response to "Median of Continuous Random Variable 1 2 Minimized"
Post a Comment